

Contents
The 2 percent rule says that any trade in a portfolio should not lose more than 2% of that portfolio.
Who came up with that 2% rule? No, it is not me.
The rule is generally accepted and can be found on Investopedia.
But the origin of this rule is little known.
I’ve also heard this rule mentioned by many other investing educators.
One of which was from a talk by David Paul
In that video, he proposed a game known as flip the coin, which we all know has a 50-50 chance of winning.
This special game has the rule that if you win, you will win twice as much as your risk.
So if you were to risk $5 and win, you would win $10 more to add to your pot.
If you were to risk $5 and lose, you would lose your $5.
Every trader will want to play this game because it has a statistically positive expectancy of winning in the long run.
The question is,
For your bet size, how many dollars do you want to bet, assuming that you have $100, to begin with?
He asked the live audience that question, and a lady said she would bet $40 on each bet.
That seems a bit high to me.
And David Paul would agree.
He says that clusters of losses can occur more frequently than most people realize.
Just go to the casino to look at the electronic display of the results of how often the roulette ball falls on a red square versus a black square.
You will see a cluster of reds and a cluster of blacks.
Instead of going to the casino, we can simulate coin flip results in Excel.
A “0” in column D means we lost the coin flip. A “1” in column D means we won the coin flip.
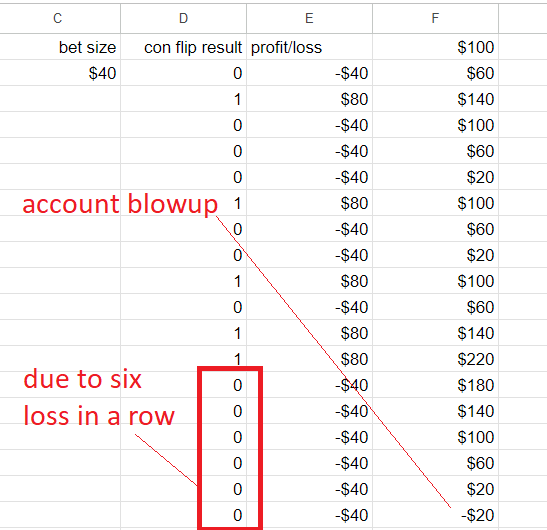
After several tries, it was not too long before I came across the above result, which had six losses in a row.
If you flip the coin enough times, you will get six heads in a row and six tails in a row.
Statistically, the chance of getting six losses in a row in a coin flip is:
0.5 x 0.5 x 0.5 x 0.5 x 0.5 x 0.5 = 1.6%
But you don’t need to lose six times in a row to have an account blow up.
Here is a sample run where a potential trader can lose the entire account balance just because four losses in a row came early in the run,

The chances of having four losses in a row in a 50-50 chance game are:
0.5 x 0.5 x 0.5 x 0.5 = 6.25%
What if 65 traders play this game, and each game consists of 100 flips of the coin?
Simulating this in Excel, where $40 was placed on each bet of the coin flip, we get the following account balance result in the end:
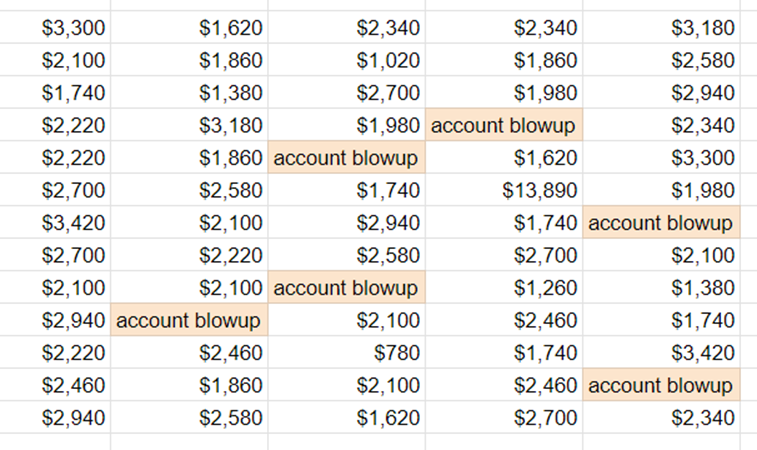
Most traders will indeed make money because this is a positive expectancy game.
This game gives the trader an advantage.
But do you want to be those six traders that were unlucky enough to have an account blow up where the account balance went negative?
This is where the 2% rule comes in.
By risking only 2% of the account per trade, the trader can withstand the cluster of losses that would wipe out the account.
That is not to say that it would prevent an account from blowing up.
There are no guarantees in trading.
It just means that it is statistically highly unlikely to have an account blow up if you risk only 2% per trade.
How unlikely?
Simulating the same game in Excel – this time using a bet size of $2 instead of $40.
Below is the result for 65 traders.
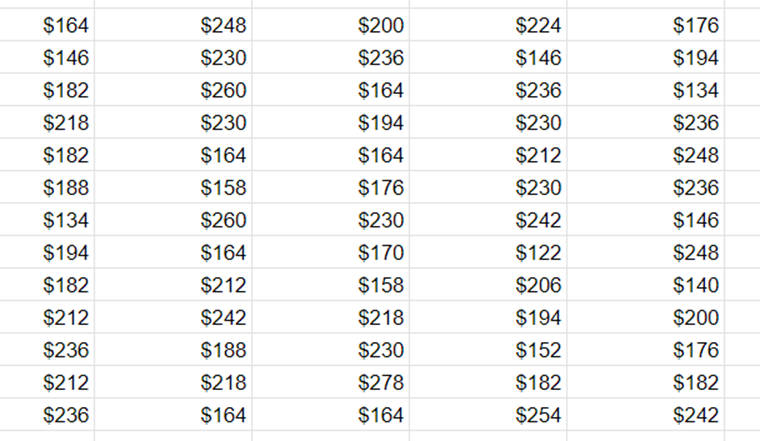
We see that not one of the 65 traders had an account blow-up.
In fact, everyone made money.
Every account balance was higher than the $100 that it started with.
Admittedly, it is not a lot of money as compared to before.
The amount of gain is much smaller than before since we use a smaller bet size.
In order to have an account blow up with a bet size of $2, a trader would have to lose this positive expectancy game 50 times in a row at the start of a run.
The chances of getting 50 losses in a row in a 50-50% chance game are 0.00000000000008882%
Some may feel that a 2% bet size is too conservative.
Again, I don’t know who came up with the 2% rule. Probably some mathematician.
It is up to the individual trader to decide their own level of risk.
Some may want to risk 10% of their portfolio on one trade with the idea of risking more in order to win more.
You need ten losses in a row to lose the entire account, risking 10% per trade.
The chances of 10 losses in a row where each trade has a 50-50% chance of winning are:
0.5 x 0.5 x 0.5 x 0.5 x 0.5 x 0.5 x 0.5 x 0.5 x 0.5 x 0.5 = 0.1%
If you risk 20% per trade, you only need five losses in a row to blow up.
The chances of 5 losses in a row are 3.12%
That may seem small. However, 3.12% is one chance out of 32.
Think in another way, play long enough with at least 32 trades.
You would see a cluster of 5 losers in a row.
If those five losers came after you had accumulated some gain, it would not wipe you out.
But if those five losers came in the beginning, and you are trading with a bet size of 20% of your portfolio, then it would drain the account to zero.
Stock Example
Let’s say that you are bullish on Salesforce (CRM), which is currently trading at $185.
You want to ut a stop-loss order at a price below the recent low of $170.
How many shares can you buy if you have a portfolio size of $10,000 and you want to conform to the 2% rule?
The max loss per share is $15.
Perhaps it is going to be a little bit more if the stock gaps overnight, etc.
But we are not going to worry about that.
2% of $10,000 is $200.
How many times $15 can you lose before hitting $200? Answer 200/15 = 13.3. So you can buy 13 shares.
If you took the stop loss on those 40 shares, you would lose $195, under the maximum loss per trade allowed for by the 2% rule.
Long Call Example
Suppose you want to buy call options instead of buying shares.
How many contracts can you buy while conforming to the 2% rule?
The call option with a strike price of $200 that is expiring 27 days out is selling at a mid-price of $1.75.
This $1.75 price quoted is a per-share price.
Since one contract controls 100 shares, it would cost $175 to buy one contract of the call option.
If you plan to hold the call option to expiration, it is possible to lose the entire $175 value of the option.
Since the 2% rule says that you can only lose $200 of the $10,000 portfolio size, you can only buy one contract.
Bull Put Spread
Continuing with the same example, suppose we decide to sell a $165/$160 bull put spread on CRM, which gives you a credit of $49 per contract.
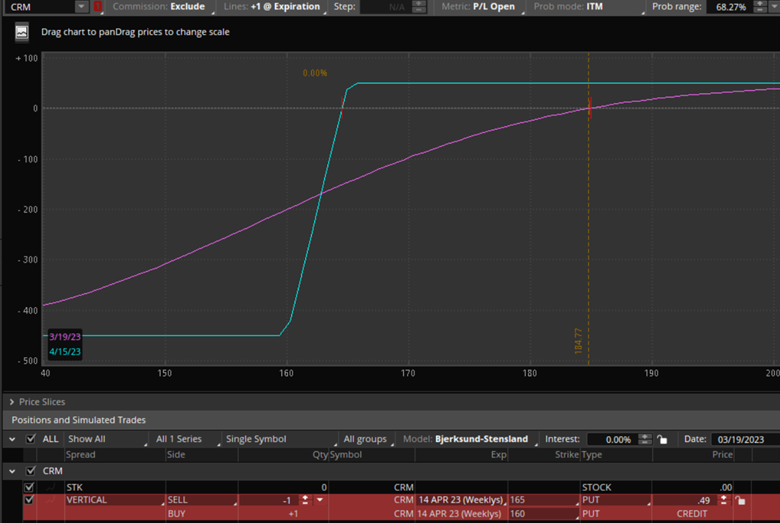
Further, suppose that your trade plan is to take profit at 80% of the max profit (in this case, $39).
And the plan says to exit the trade if the loss exceeds two times the initial credit (in this case, $98).
While the risk graph shows that this credit spread has a max loss of $450, you will never realize this loss if you follow your trade plan and exit at the $98 loss limit.
Your effective risk in the trade is $98 for the purpose of the 2% rule calculation.
So you can trade two such credit spreads, with a potential loss of $196 if trade triggers your planned loss limit.
This assumes that you are able to diligently exit at the loss trigger.
Also, understand that the risk may be slightly more than 2% to allow for some leeway in the event that the loss may exceed $98 due to fill issues or not being able to get to your machine, gaps, etc.
Butterfly Example
Suppose you have a larger portfolio size of $100,000 and are trading a butterfly strategy where your trade plan says to exit the trade if you have a loss of 15% of the margin.
The margin on a one-lot butterfly is $1000.
How many contracts of this butterfly can you trade in this portfolio while still conforming to the 2% rule?
15% of $1000 is $150.
$150 is the max loss per butterfly if traders are able to exit as planned.
2% of $100,000 is $2000.
How many $150 are in $2000?
You can trade 13 contracts in this butterfly trade.
Next question: 13 is an odd lot size.
Suppose you decide not to conform to the 2% rule and decide to trade a 20-lot butterfly instead.
What is the risk of this trade in terms of the percentage of the portfolio?
A 20-lot butterfly can lose up to 20x$150 = $3000.
So this 20-lot butterfly trade is risking 3% of the $100,000 portfolio’s value.
Conclusion
You have seen various examples of how to apply the 2% rule.
I hope this article didn’t sound like a math textbook.
Another factor to consider is correlated trades.
Although the rule says that one trade is allowed to risk 2% of the portfolio, if you have ten correlated trades, you are effectively risking 20% of the portfolio.
Correlated trades mean that if one trade fails, the other trade will behave in the same way.
For example, buying Pepsi (PEP) and Coca-cola (KO) stocks may be two separate trades. But they often move the same way. So if one loses, the other loses as well.
Hence, there are benefits to having a portfolio using a set of diverse strategies.
We hope you enjoyed this article about the 2 percent rule.
If you have any questions, please send an email or leave a comment below.
Trade safe!
Disclaimer: The information above is for educational purposes only and should not be treated as investment advice. The strategy presented would not be suitable for investors who are not familiar with exchange traded options. Any readers interested in this strategy should do their own research and seek advice from a licensed financial adviser.










